Hands On! Magazine

Building Solutions for Algebra Readiness — Hands On! Winter 2018

By Maria L. Blanton and Angela Murphy Gardiner, TERC; Eric Knuth, University of Texas Austin; Ana Stephens, University of Wisconsin Madison; Despina Stylianou, City College of New York; Rena Stroud, Merrimack College

Love it or hate it, almost everyone has had a brush with algebra at some point in school mathematics. For too many students, these experiences have left them with a sense of failure and even dread.

Schoenfeld wisely observed that algebra has become a gatekeeper, “an academic passport for passage into virtually every avenue of the job market and every street of schooling,” that has pushed students out of opportunities in STEM-related careers (Schoenfield, 1995, p. 11). This reality has particularly impacted students in underrepresented groups (Moses & Cobb, 2001; Museus, Palmer, Davis, & Maramba, 2011).

It is important to understand how algebra developed into an obstacle for so many students. Historically, the focus in elementary grades on arithmetic—particularly, computational work—was followed by an abrupt and largely shallow treatment of algebra in secondary grades (Kaput, 2008). Simply put, students did not have the time or conceptual space to develop insights into the deeper, more abstract elements of algebra, and their (at best) superficial knowledge of algebra resulted in widespread failure in school mathematics (Stigler, Gonzales, Kawanaka, Knoll, & Serrano, 1999). In recent decades, the breakdown of this approach led to calls for radical change in teaching and learning algebra in the US to a grades K–12 approach, in which the development of algebraic thinking would begin in elementary grades in ways that would build naturally on children’s informal intuitions about structure and relationships.

The prospect of this shift raised significant questions. What would such an approach look like in the elementary grades? Would it amount to “pre-algebra” ideas repackaged for young children? Would young children even be capable of thinking in ways that have traditionally been viewed as possible only for older students? What impact, if any, would such an approach have on students’ algebra readiness for secondary grades? And, given that elementary teachers would be at the forefront of reform in algebra education, how should they be prepared to build authentic algebra learning environments that would not reinforce past student failures? A number of researchers have worked to address these questions over the past several decades,1 and while open questions remain, we currently have a much better picture of the potential for early algebra2 to alleviate algebra’s gatekeeper status.

What Is (Early) Algebra?

Likely, one of the most important questions in reconceptualizing algebra for elementary grades is “What is the ‘algebra’ we want young children to learn?” Early algebra is not algebra early (Carraher, Schliemann, & Schwartz, 2008). In particular, the focus of early algebra is not on manipulating algebraic equations and expressions, too often a major component of typical algebra classes in secondary school mathematics. Project LEAP3 takes a view of early algebra as a set of core thinking practices (Kaput, 2008): generalizing, representing, justifying, and reasoning with mathematical structure and relationships (Blanton, Brizuela et al., 2018). Noticing mathematical structure and relationships—such as the Commutative Property of Addition or a function that depicts how two quantities vary in relation to each other—and representing these generalizations through words, algebraic notation, tables, graphs, or pictures, is often viewed as the heart of algebraic thinking (Cooper & Warren, 2011; Kaput, Blanton, & Moreno, 2008). However, the two practices of justifying (or refuting) generalized claims and reasoning with generalizations to build new mathematical knowledge are equally important components of algebraic thinking. For example, students might build arguments as to why the sum of two odd numbers is even and then use the claim that “the sum of two odd numbers is even” to reason in new ways about the sum of three odd numbers. They might use properties of operations they notice, such as commutativity or associativity, to reason in more strategic ways in computational work. All these practices provide an important framework that lays the foundation for algebra.

The LEAP Curriculum

Over the last decade, our team4 has worked to integrate these core algebraic thinking practices into the design of an early algebra curriculum for grades K–5 and to examine its impact on children’s algebra understanding and their algebra readiness for middle grades. Currently, we have completed the LEAP curriculum for grades 3–5, a sequence of 18 lessons for each grade level, along with grade-level assessments to measure students’ learning as they progress through the sequence.

The curriculum engages students in the four core algebraic thinking practices across different important areas of mathematical content, using increasingly sophisticated ideas, concepts, and representations. The design of the curriculum uses a learning progressions approach (Clements & Sarama, 2004) that incorporates empirical, classroom-based research on levels of growth in children’s thinking about algebraic thinking practices and concepts. Learning progressions, which are increasingly endorsed for their potential to inform the design of coherent standards, curricula, assessment, and instruction (CCSSI, 2010; Daro, Mosher, & Corcoran, 2011), provide an important research paradigm for our work.

Lessons begin with a brief “Jumpstart” to review previous concepts or prompt students’ thinking about new concepts, then transition into small-group investigations in which students explore concepts that engage them in algebraic thinking practices. They conclude with a whole-group discussion of students’ findings and a “Review and Discuss” that serves as a formative assessment. Across grades 3–5, the curriculum develops increasingly sophisticated understandings of algebraic concepts and practices, emphasizing the development of meaning for algebraic ideas by engaging students in explaining and justifying their thinking, both orally and in writing.

Can Children Think Algebraically?

Through experimental studies, we have found that students who are taught the LEAP curriculum as part of their regular math instruction significantly outperform students who receive only regular, arithmetic-focused instruction on growth in understanding of core algebraic concepts and practices (Blanton et al., 2017; Blanton et al., 2018). LEAP students are significantly more able to interpret the equal sign as a relational symbol, recognize properties of operations and represent them with variable notation, build arguments for mathematical claims that are increasingly general and more sophisticated than arguments that test numerical examples, recognize unknown quantities in mathematical situations and represent them as algebraic expressions, and generalize functional relationships and represent them with words and variable notation. Surprisingly, we have also found that students are more successful in representing function rules with variable notation than with their own words, underscoring the argument that variable notation can be an important tool in even young children’s algebraic reasoning.

Consider the following example of growth in students’ ability to generalize and represent relationships—two core algebraic thinking practices. In the “Brady Problem” (see Figure 2), students were asked a variety of questions, including whether they could find a relationship between the number of desks and the number of students that could be seated at the desks and to represent this relationship with words and variable notation. Table 1, which records one student’s responses across grades 3–5, shows increasingly sophisticated ideas that grow from not recognizing a pattern or relationship at the beginning of third grade, to a clear understanding of a correspondence functional relationship in fifth grade. Our most compelling finding, however, is that the effectiveness of the LEAP curriculum also holds for those students who come from disadvantaged and demographically diverse backgrounds (Blanton et al., 2018): LEAP students from low SES (socioeconomic status) and demographically diverse schools significantly outperform their peers in similar schools where only the regular arithmetic curriculum is taught. For us, this shows great promise in LEAP’s potential to ameliorate algebra’s gatekeeper effect and broaden the STEM pipeline to include underrepresented groups.

Figure 2. The Brady Problem.

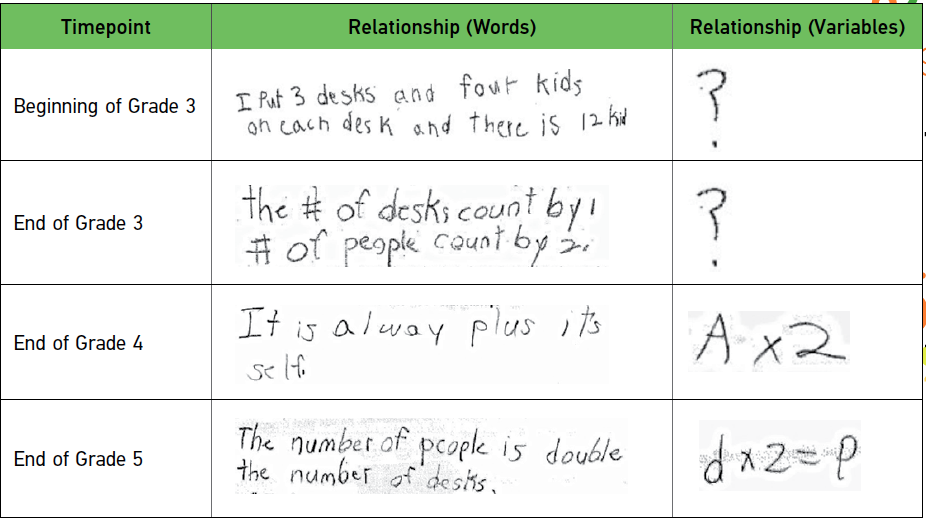
Table 1. One student’s response to the Brady Problem across grades 3–5.

We are optimistic that curricula such as LEAP can continue to change the way students learn algebra and lead to more opportunities for success in mathematics for all students. Currently, we are working on two core goals: first, expand LEAP into grades K–2; and second, identify and test design principles that address the needs of students with learning difficulties and differences and that encourage culturally responsive teaching. The design of a comprehensive, grades K–5 approach to teaching and learning algebra that addresses the needs of all learners will, in our view, make an important contribution to developing children’s algebra readiness.

ENDNOTES

¹ For an overview of the state of research on children’s algebraic thinking, see Cai & Knuth (2011); Carraher & Schliemann (2007); Kaput, Carraher, & Blanton (2008); and Stephens, Ellis, Blanton, & Brizuela (2017).

² By early algebra, we mean algebraic thinking in elementary grades.

³ The LEAP (Learning through an Early Algebra Progression) moniker is based on the use of a learning progressions approach in the curriculum’s design.

⁴ The Project LEAP team is a collaboration among researchers at TERC, the University of Wisconsin Madison, the University of Texas Austin, City College of New York, and Merrimack College.

ACKNOWLEDGEMENT

The research reported here was supported in part by the National Science Foundation under DRK-12 awards 1219605 and 1219606 and by the Institute of Education Sciences, U.S. Department of Education, through grant R305A140092. Any opinions, findings, and conclusions or recommendations expressed are those of the authors and do not represent the views of the National Science Foundation or of the Institute of Education Sciences or the U.S. Department of Education.

REFERENCES

Blanton, M., Isler, I., Gardiner, A., Stephens, A., Knuth, E., & Stroud, R, & Strachota, S. (2017). Growth in children’s algebraic thinking: A grades 3–5 early algebra intervention. Paper presented at the National Council of Teachers of Mathematics Research Conference, San Antonio, TX.

Blanton, M., Brizuela, B., Stephens, A., Knuth, E., Isler, I., Gardiner, A., Stroud, R., Fonger, N., & Stylianou, D. (2018). Implementing a framework for early algebra. In C. Kieran (Ed.), Teaching and learning algebraic thinking with 5- to 12-year-olds: The global evolution of an emerging field of research and practice. (pp. 27-49). Hamburg, Germany: Springer International Publishing.

Blanton, M., Stroud, R., Stephens, A., Gardiner, A., Knuth, E., Stylianou, D. (2018). An Early Algebra Intervention for Upper Elementary Grades. Paper presented at the National Council of Teachers of Mathematics Research Conference as part of the Symposium Rigorous Research to Improve Learning in Elementary Math for All Students, Washington, DC.

Cai, J., & Knuth, E. (Eds.). (2011). Early algebraization: A global dialogue from multiple perspectives. Heidelberg, Germany: Springer.

Carraher, D. W., & Schliemann, A. D. (2007). Early algebra and algebraic reasoning. In F. K. Lester, Jr. (Ed.), Second handbook of research on mathematics teaching and learning (Vol. 2, pp. 669–705). Charlotte, NC: Information Age Publishing.

Carraher, D. W., Schliemann, A. D., & Schwartz, J. L. (2008). Early algebra is not the same as algebra early. In J. Kaput, D. Carraher & M. Blanton (Eds.), Algebra in the early grades (pp. 235-272). Mahwah, NJ: Lawrence Erlbaum Associates.

Clements, D. H. & Sarama, J. (2004). Learning trajectories in mathematics education. Mathematical Thinking and Learning, 6(2), 81-89.

Cooper, T., & Warren, E. (2011). Years 2 to 6 students’ ability to generalize: Models, representations, and theory for teaching and learning. In J. Cai & E. Knuth (Eds.), Early algebraization: A global dialogue from multiple perspectives (pp. 187-214). Heidelberg, Germany: Springer.

Daro, P., Mosher, F., & Corcoran, T. (2011). Learning trajectories in mathematics: A foundation for standards, curriculum, assessment, and instruction. Retrieved from
http://www.cpre.org.

Kaput, J. (2008). What is algebra? What is algebraic reasoning? In J. Kaput, D. Carraher, & M. Blanton (Eds.), Algebra in the early grades. Mahwah, NJ: Lawrence Erlbaum Associates/Taylor & Francis Group.

Kaput, J. J., Blanton, M. L., & Moreno, L. (2008). Algebra from a symbolization point of view. In J. J. Kaput, D. W. Carraher, & M. L. Blanton (Eds.), Algebra in the early grades (pp. 19–55). New York: Lawrence Erlbaum/Taylor & Francis Group & National Council of Teachers of Mathematics.

Moses, R. P., & Cobb, C. E. (2001). Radical equations: Math literacy and civil rights. Boston: Beacon Press.

Museus, S., Palmer, R. T., Davis, R. J., & Maramba, D. C. (2011). Racial and Ethnic Minority Students’ Success in STEM Education. Hoboken, NJ: Jossey-Bass.

Schoenfeld, A. H., (1995). Is thinking about ‘Algebra’ a misdirection? In C. Lacampagne, W. Blair & J. Kaput (Eds)., The Algebra Colloquium. Volume 2: Working Group Papers (pp. 83-86). Washington, DC: US Department of Education, Office of Educational Research and Improvement.

Stephens, A. C., Ellis, A. B., Blanton, M., & Brizuela, B. M. (2017). Algebraic thinking in the elementary and middle grades. In J. Cai (Ed.), Third Handbook of Research on Mathematics Teaching and Learning (pp. 386-420). Reston, VA: National Council of Teachers of Mathematics.

Stigler, J.W., Gonzales, P., Kawanaka, T., Knoll, S., & Serrano, A. (1999). The TIMSS videotape classroom study: Methods and findings from an exploratory research project on eighth-grade mathematics instruction in Germany, Japan, and the United States. (NCES1999-074). U.S. Department of Education. Washington, DC: National Center for Education Statistics.