Blog

Will This Be on the Test? (May 2025)

by Aren Lew

---

Welcome to the latest installment of our monthly series, “Will This Be on the Test?” Each month, we’ll feature a new question similar to something adult learners might see on a high school equivalency test and a discussion of how one might go about tackling the problem conceptually.

---

Welcome back to our continuing exploration of how to bring real conceptual reasoning to questions students might encounter on a standardized test.

Did you know that the EMPower™ series includes a set of test practice questions with each lesson? This month let’s take a look at a question Lesson 1 from the EMPower™ title Split It Up: More Fractions, Decimals, and Percents.

One question worth asking with this problem is whether or not you would use a calculator. A calculator can feel like a lifeline when you don’t know what to do, but it is not always as helpful as it seems. I had the opportunity recently to sit with a student who was working on this task. They saw the word “each” in the problem and figured it meant that they should multiply the numbers, which they did easily on the calculator. When they did not find their answer among the answer choices, they had no other ways of moving forward. If their first direction had been to make sense instead of looking for key words and reaching for a calculator, it might have gone very differently.

Here are some possible approaches that start with making sense:

1. Estimate. Estimation is mathematically valuable in so many ways. Many times in the real world, an approximate answer is good enough and easier to get to. Even on the test, estimation can get a student partway or all the way to a correct answer. For evidence, see about 90% of the blogs in this series (that’s an estimate!). In this case, estimation is baked into the question since the goal is to find about how much each piece of gum costs. Here’s one way to estimate:

2. Play “What if?”. This strategy works nicely as a complement to estimation. A student can look at the answer choices and ask which are reasonable. What if the pieces of gum cost 80¢ each? Would that come to a total of close to 89¢ for ten pieces? Probably not because with only one piece the cost is already close to 89¢. Having started with an estimate, a student might be wondering whether 8¢ or 9¢ is a better answer since both are a little less than a dime. Finding the cost of 10 items at 8 or 9 cents each is accessible to many students without a calculator, but if the pack had contained a less friendly number of pieces, like 7 or 13, picking up a calculator to do a quick multiplication could save a student time and stress in the moment. The important thing is that the calculator would be supporting reasoning, not replacing it. At 8¢ each, the pack of gum comes to 80¢ total. At 9¢ each, it comes to 90¢. 

3. Use a bar model. While it is certainly faster to recognize this as a division situation, it’s not necessary to name the operation to be able to find an answer that makes sense. Bar models can help us figure out what makes sense to do by making the information in the task visual. A bar model for this task could look like this:

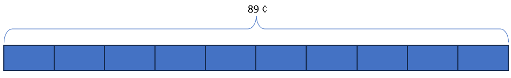
How do you see the information presented in the task represented in this model? A student might look at this and recognize that a way to solve it is to divide 89 into 10 equal pieces and that that can be done on a calculator (if one is allowed). However, this model can also be a frame for guessing and checking: Will it come out close if I put 4¢ in each box? What about 8¢ or 9¢? Which number of pennies gets me closest? A student who recognizes this as a matter of parceling out the 89 pennies as evenly as possible into 10 equal boxes but doesn’t have a calculator or a quick strategy for dividing could count the 89 pennies by putting tally marks in the boxes like this:

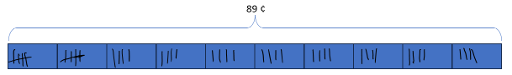
This model is incomplete, showing only 42 tally marks so far. How would you continue it?

(Ultimately, this approach will lead to not having enough pennies to put one more in each box. One option in that case is to just keep parceling out the pennies until they are gone and not have the same number in each box. Try drawing this out yourself and see if it gives you an idea of whether the answer to the question of how much each piece of gum costs is closer to 8¢ or 9¢.)

4. Use a number line for rounding. As I was sitting with the student working on this task, another student leaned over and suggested that they should divide instead of multiplying, which the student did on the calculator. Then they had an answer, 8.9. At this point, they decided the answer must be (B) 8¢. Even with a suggestion of what operation to do and with a calculator, the student still arrived at an incorrect answer because they weren’t relying on their own reasoning. Instead, they accepted another person’s suggestion, used the calculator and stopped as soon as they had an answer that was among the choices. Also, using a calculator to divide brought decimals into the question, and this student might have been more comfortable using one of the other strategies that involved whole number reasoning. They now needed a tool to help them ask and reason about the question of whether 8.9¢ was closer to 8¢ or to 9¢. Using a number line to connect decimals to visual tenths can help here.

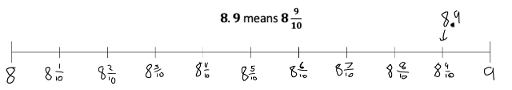
A thought about currency and symbols: I ask myself to what degree familiarity with U.S. currency is an advantage in this question; both students who have immigrated to the U.S. and younger adults who grew up here may not have a lot of experience with U.S. coins. Context matters, and an unfamiliar context imposes an additional cognitive load. One thing we can do to mitigate potential issues to practice recognizing that units are units even if we’re not familiar with them. Suppose the question had asked, “A 10-piece pack of gum costs 89Ω. Each piece of gum costs about…” Despite not knowing the meaning of Ω, we can deduce that it’s a unit of money because the question is about cost. If a student can look past not being familiar with the unit, they can still reason with any of the strategies above because the answer choices are given in the same unit, whatever it is. Building familiarity with the units used in this country is important, context matters, and students can also strengthen their ability to navigate situations where the units are unfamiliar.

---