Blog

Will This Be on the Test? (September 2022)

by Sarah Lonberg-Lew

---

Welcome to the latest installment of our monthly series, “Will This Be on the Test?” Each month, we’ll feature a new question similar to something adult learners might see on a high school equivalency test and a discussion of how one might go about tackling the problem conceptually.

---

After a summer hiatus, Will This Be on the Test is back! Welcome back to our continuing exploration of how to bring real conceptual reasoning to questions students might encounter on a standardized test. This month’s question is inspired by a discovery I made while pursuing some new learning of my own. I have been trying to resurrect and build on the Spanish I learned in college and as part of my studies, I have been streaming TV shows and movies in Spanish. I discovered that some streaming services will let me change the speed at which the video is played, slowing down the dialogue so I can understand it better. This led me to a very interesting question which I’ve adapted here into a test-like format:  

As with many contextual math tasks, it is not obvious which domain this question fits in. Is it a question about percents? About algebra? About proportional reasoning? How are you supposed to know whether you are supposed to write an equation, do a calculation, or draw a diagram? The questions on “the test” and math questions we encounter in real life don’t come at the end of a chapter on the specific math content needed to answer them, and that can make it hard to figure out which is the “right” procedure to apply. Not to worry, however, because as with most math that shows up in the real world, there isn’t one “right” procedure or way to approach it. Here are some approaches a student who is accustomed to thinking flexibly and creatively might take:

1. Make sense of the problem. This is always an important first step in problem-solving. It’s so important that it is the beginning of Standard for Mathematical Practice number one: Make sense of problems and persevere in solving them. For a question like this, it is worth it to take a minute to think about what the question means and what an answer would look like. Specifically, what impact does slowing down a show have on the time it takes to play it? Students who do not pause to make sense of the problem may fall victim to a distracting answer choice if they resort to number grabbing and quickly find 75% of 30 minutes. Students who pause to think about the real context will realize that slowing down a show will make it take longer to watch.

2. Try some simpler numbers. Slowing the speed to 75% is tough to reckon with, but what about slowing it to a friendlier 50%? If Isabel watches the show at half speed, how long will the whole show take? If an intuitive answer doesn’t show up, shifting contexts might help. For example, if it normally takes me 20 minutes to get home from work but traffic slows me down to half my usual speed, how long will it take me to get home? (It might also help to ask: If I am driving at half the speed, what fraction of my commute will I have covered in my normal commuting time?) How does trying it with simpler numbers help when those aren’t the numbers in the problem? It might help a student look for and make use of structure…

3. Look for and make use of structure. Trying simpler numbers is a way on getting a handle on the structure of the situation. A table using simpler changes to the speed might help. Can you use your understanding of the structure to complete the empty cells?

Fraction of speed	Effect on time
Half speed	It takes twice as long
Quarter speed
Double speed
	It takes one-third as long

How can you use your understanding of what happens when you slow down or speed up by simpler numbers to make sense of what happens when you slow the show to 75% or ¾ of its normal speed? (Hint: How is ¾ speed related to ¼ speed?)

4. Look for and make sense of structure II. Another way of thinking about the effect of slowing down the show is to think about how much of the show you will have watched in a given amount of time. A colleague I shared this task with figured that slowing down the show meant she’d see less of the show in the same amount of time and reasoned this way:

How long I’ve watched	How much of the show I’ve seen
1 minute	45 seconds (75% of 1 minute)
4 minutes	3 minutes
8 minutes	6 minutes
12 minutes	9 minutes
…	…
??? minutes	30 minutes

5. Visualize the progress bar. Along similar lines to the reasoning in the approach above, a student might reason that after 30 minutes, they would only have watched 75% of the show. (At normal speed, you’d watch 100% of the show in 30 minutes, so at 75% speed, you’d watch 75% of the show in the same amount of time.)

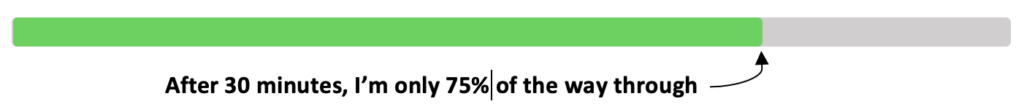
How might you mark up the diagram above to help you reason about the total time?

Did you come up with a strategy that wasn’t in this blog? The list here is definitely not exhaustive!

By the way, did we ever answer the question of which domain this task belongs in? I hope that after having tried it yourself and having read through the approaches above, you’ll agree with me that it doesn’t neatly fit in a single domain. You might have found yourself reasoning proportionally (domain of Ratios and Proportional Relationships) or algebraically (domains of Operations and Algebraic Thinking and Expressions and Equations). Or you might have been thinking about fractions and operations (domain of Numbers and Operations – Fractions). Or maybe your thinking encompassed more than one domain. Such is often the case with the math we use in the real world and even with the math we do for “the test.” We prepare our students for mathematical success when we embrace the connections between domains and the messiness of being open to different approaches.

I really enjoyed thinking about this question when it arose for me. When I shared it with some friends and colleagues, they all reacted by saying things like, “What an interesting question! I can’t wait to try to figure it out!” and “Don’t tell me the answer, I want to think about it!” Problem solving can be an enjoyable challenge when you have the confidence to approach it as such. Being open to and celebrating multiple approaches and ways of reasoning in your classroom can help students shift their perception of math from a frustrating ordeal to an enjoyable challenge.  

---