Blog

Will This Be on the Test? (December 2020)

by Sarah Lonberg-Lew

Welcome to the fourth installment of our monthly series, “Will This Be on the Test?” Each month, we’ll feature a new question similar to something adult learners might see on a high school equivalency test and a discussion of how one might go about tackling the problem conceptually. (You can find earlier installments as you scroll down the blog.)

This month let’s dive into one of my favorite types of problem to see on a test – an algebra story problem. I love this kind of problem because I think it’s accessible to almost anyone who has the confidence to play with it. The context makes it accessible. I always encourage my students to look for those problems where there is a story they can understand. With that toehold, they often can figure it out. What about you? Can you figure out this month’s challenge without using procedures you memorized in algebra class?

When you’ve given yourself enough time to really explore, read on to see some possible ways students might approach this problem using conceptual understanding and visuals.

1.A student might reason (proportionally!) that if 4 pencils and 4 erasers cost $3.20, half as many pencils and erasers would cost half as much. That means 2 pencils and 2 erasers cost $1.60. Now the student might compare the cost of 2 pencils and 2 erasers to the cost of 3 pencils and 2 erasers (a picture really helps):

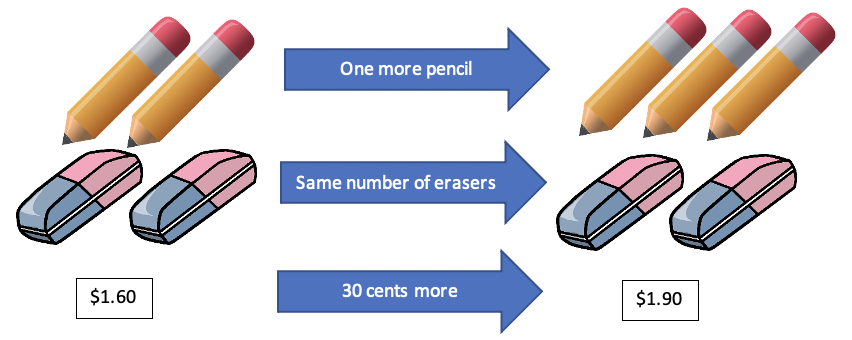
If the only difference between the two pictures is a single pencil and the difference in price is 30 cents, the pencil must cost 30 cents! But wait – the question didn’t ask for the cost of a pencil! This is a great start, but it’s always important to make sure you’ve finished the problem, especially on a test. Once they know the cost of a pencil, the student would have several options for figuring out the cost of an eraser.

2. Alternatively, a student might reason that they could figure out what 1 pencil and 1 eraser cost together by dividing the cost of 4 pencils and 4 erasers by 4. The combined cost of a single pencil and eraser is $0.80. Knowing what they cost together means that you can find the cost of one if you know the cost of the other. This paves the way for a pretty efficient guess-and-check.

• GUESS 1: If the eraser costs $0.10, then the pencil costs $0.70. This means that the cost of Jason’s purchase would be 3($0.70) + 2($0.10) = $2.40. That’s way too much. (Jason only spent $1.60.)
• GUESS 2: Since the first guess was way off, the student might jump to answer choice (c) or (d). Let’s try c. If an eraser costs $0.30, then the pencil would cost $0.50. This means that the cost of Jason’s purchase would be 3($0.50) + 2($0.30) = $2.10. That’s closer, but still too much.
• GUESS 3: At this point there are only two possible answer choices. Trying either one will get a student to the correct answer, either directly or by eliminating the other possibility.

3.Speaking of guess-and-check, that’s almost always a workable approach to a situation like this. Even if a student can’t write an equation to find the cost of a pencil if they know the cost of an eraser, they can probably work it out by thinking about the real items. For example, a student might guess that the eraser costs $0.20. Then they might look at Jason’s purchase: 3 pencils and 2 erasers cost $1.60. If the erasers are $0.20 each, they would account for $0.40 of the cost, leaving the remaining $1.20 as the cost of 3 pencils. This means that the pencils must cost $0.40 each. Now the student could check to see if these two costs work out in the larger purchase. 4 pencils at $0.40 each and 4 erasers at $0.20 each would total $2.40. This doesn’t match Irita’s cost, so the guess was wrong.

A student might even keep track of their thinking in a table like this (based on starting with Jason’s purchase and then checking with Irita’s purchase):

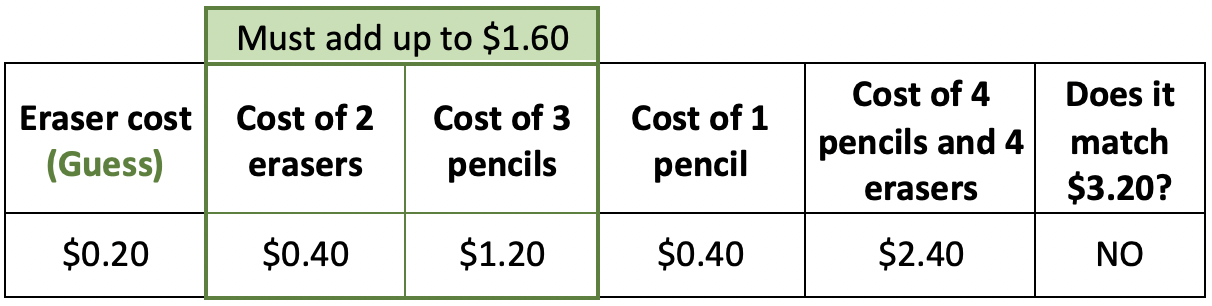
That was a lot of work to eliminate just one answer choice, but remember that there will be questions on the test that are not within students’ reach at all. It’s okay to spend some extra time on a problem they have a good chance of getting right. (Also, try not to worry about the fact that students will probably not make a neat table with everything labeled precisely when they are under pressure in a test situation. Tables are an excellent way to keep your thinking organized and if students practice with and feel confident using them in your class, they will be more likely to make use of a quickly sketched table in a test situation. Even a reduced table where students just keep track of their guesses and the cost of 4 pencils and 4 erasers will help them navigate a complicated situation like this.)

As teachers, we are charged with getting our students to pass the test and that is often what’s driving our students as well. But focusing on the test often brings with it the mistaken idea that there is one right way to solve each problem. A student who sees this problem as a prompt to remember and apply a procedure may freeze up and miss the chance to score some points. On the other hand, a student who values their own ability to reason about quantities and relationships, especially in the familiar context of money, may make sense of this in their own creative way. Teaching students to reason flexibly and creatively, to be problem solvers, and to value their own prior knowledge and experience will not only prepare them for the test but also help them grow as numerate citizens and critical thinkers.

---