Will This Be on the Test? (September 2020)
by Sarah Lonberg-Lew
Welcome to the first installment of our new monthly series, “Will This Be on the Test?” Each month, we’ll feature a new question similar to something adult learners might see on a high school equivalency test and a discussion of how one might go about tackling the problem conceptually.
There are lots of good reasons to study math – it better prepares students for the numbers and relationships they encounter in life and at work, it builds flexible thinking and analytical skills, and students have to pass a test on it to move forward with their educational and career goals. The test looms large for students and is often their strongest motivation for coming to adult ed classes. For teachers and programs, the test occupies a place of great significance as well. Performance reviews for teachers and funding for programs may hinge on how many students pass the test and what scores they get. It’s no surprise, therefore, that directors, teachers, and students are inclined to prioritize preparing for the test over anything else. The good news is that preparing students for the test doesn’t have to be separated from deep and rich conceptual learning. In fact, deep and rich conceptual learning prepares students better than crash courses on procedures and test-taking strategies. This post is the first in a series that will look deeply at test-style questions and dig into how conceptual understanding, willingness to draw pictures, and flexible thinking can prepare students to excel on the test.
Here is an example of a question students might see on a high school equivalency (HSE) test. Before you read further, I challenge you to see how many ways you can think of to approach it. You may know a procedure to apply. Which approaches could be used that do not rely on memorized procedures? What skills and understandings do you think students really need to be able to arrive at the correct answer?
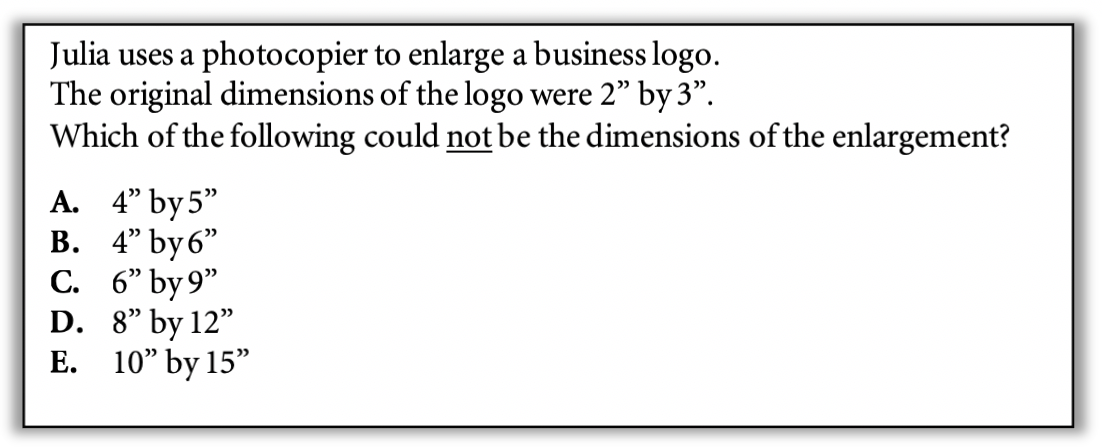
Here are some approaches students might take if they don’t have a memorized procedure:
1. A student might sketch rectangles with all the sets of dimensions including the original and eyeball them to see which one looks like it’s not similar to the rest of them.
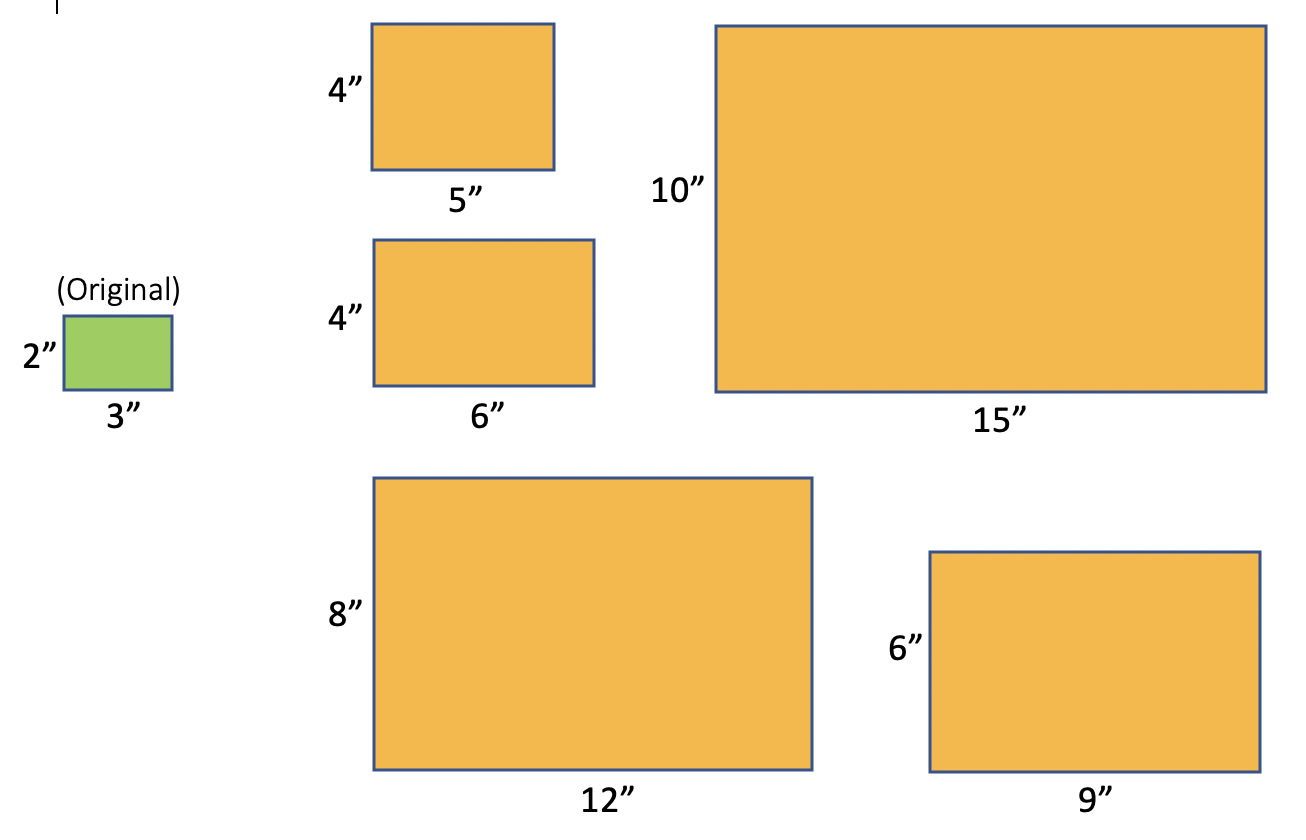
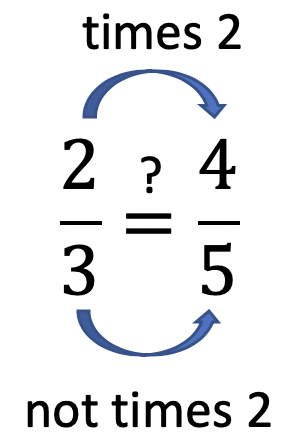
2. A student might start by drawing a picture showing a simple enlargement. The simplest way to enlarge a picture is to double both dimensions.
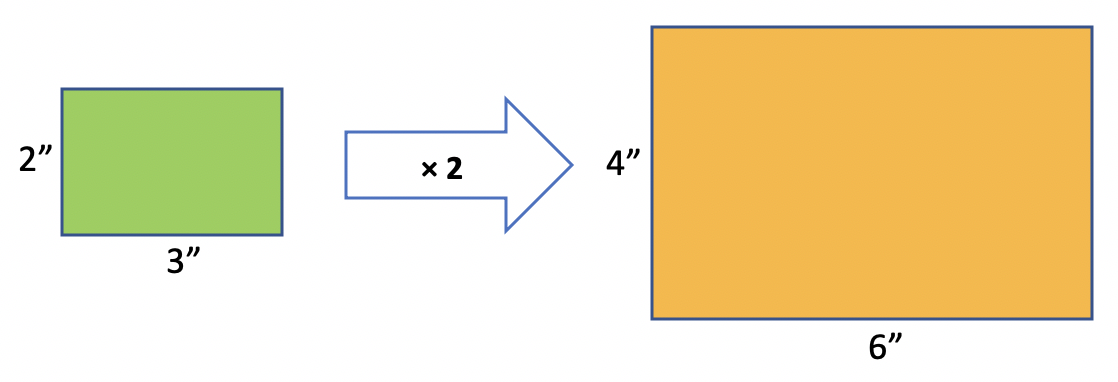
Based on this picture, a student might reason that there was only one proper enlargement with a 4” height and since doubling the dimensions gave one that is 4” by 6”, an enlargement that is 4” by 5” would not work.
3. A student might look at the ratio of the length to the width of the logo as a fraction and visually compare the fractions for each enlargement.
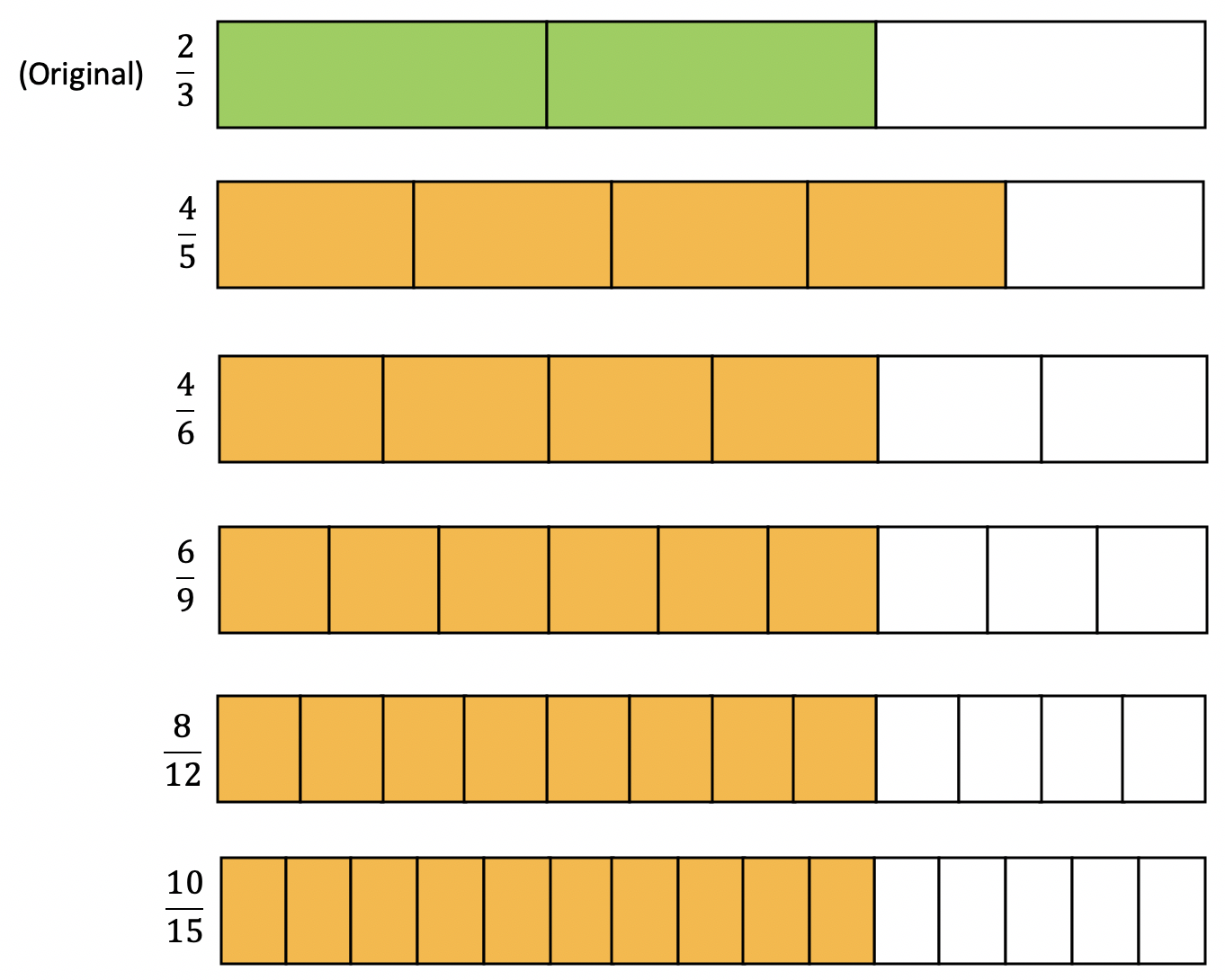
4. A student might reason that you enlarge a picture by multiplying both dimensions by the same number and notice that answer choice A is the only one where the second dimension is not a multiple of 3. If she then checked by trying to set up equal ratios, her suspicions would be confirmed.
Does this mean that instead of teaching one procedure you need to teach four different ways to approach the problem? No! The purpose of seeing all these different approaches is to understand that when students have some conceptual understanding and the confidence to apply it flexibly, they can make sense of challenging test questions even if they haven’t memorized the procedure that the question is ostensibly targeting. To tackle this question, a student does need some intuition about what it means to enlarge a picture, but even with only that, she is prepared to make sense of the problem and persevere in solving it. In fact, worrying about which memorized procedure applies to this problem can make it harder!
Giving students rich learning experiences that help them draw connections between math concepts and the real world gives them the confidence to bring their strengths to unfamiliar test questions. When students know that there isn’t one single correct approach to math, they are free to find their own strategies.

Sarah Lonberg-Lew has been teaching and tutoring math in one form or another since college. She has worked with students ranging in age from 7 to 70, but currently focuses on adult basic education and high school equivalency. Sarah’s work with the SABES Mathematics and Adult Numeracy Curriculum & Instruction PD Center at TERC includes developing and facilitating trainings and assisting programs with curriculum development. She is the treasurer for the Adult Numeracy Network.
