Rounding is NOT a Procedural Skill
by Melissa Braaten
I remember being taught rounding as a simple, mechanical procedure.
- Find the place value to the left.
- If it is a 5 or higher, round up. Otherwise, round down.
I also remember something about a balloon: hold the balloon in your hand. If you open 0, 1, 2, 3, or 4 fingers, the balloon stays put. Once you open 5 fingers, it floats up. (Quite a nice visual mnemonic).
Unfortunately, these steps and mnemonics did not prepare me to use rounding very effectively in the real world. Neither the procedure (nor the balloon) makes any reference to why 5 has this magical quality of going up, or how I decide which place value to round to when there are no instructions, or how to interpret a statement like “we are expecting about 100 participants.” This simple procedure is a distillation of many underlying concepts; if we want students to use rounding as a flexible and useful skill for navigating numerical situations in their lives, we need to unpack those concepts, make sure students understand them, and give them practice using rounding in more realistic ways.

“My dog weighs about 30 pounds.” This is the type of rounded number we encounter all the time. In class, I start my discussion of rounding with this statement, and ask students to discuss what they think the exact weight might be. This is always an interesting discussion, as we probe the boundaries of what seems reasonable and why, and discuss whether the exact weight could be over or under 30 pounds (this was hotly contested the last time we discussed it). But this type of reasoning (i.e., where did the rounded number come from?) is important. We encounter rounded numbers all the time, with no more information than the word “about” or “approximately” to indicate that some rounding has occurred. Is it reasonable for the school with 192 students to report they serve “around 200?” What if there were 162 students?
In addition, real-life rounding does not come with worksheet instructions about which benchmarks to which we should round. I gave my students the age of a woman who is 48 years and 9 months old. I asked them about how old she was. Some argued 49 years (nearest year), other argued for 50 (nearest ten), both of which seemed reasonable in the context. However, it did not seem reasonable to round to the nearest 100, which would put her age as 0!
Let’s unpack some of the mathematical concepts that underlie the idea of rounding and allow students to use it flexibly and reasonably in their daily lives.
Order: To round, students need to understand which benchmarks a given number falls between. For example, if they are rounding to the nearest dollar, they need to know that $16.34 is between $16 and $17. This gets a little tricky with higher (or decimal) place values: for example, if locating the number $214.65 between 214 and 215, between 210 and 220, or between 200 and 300, depending on the place value.
Number lines can be a helpful visual to support students who struggle to locate a number between appropriate benchmarks.
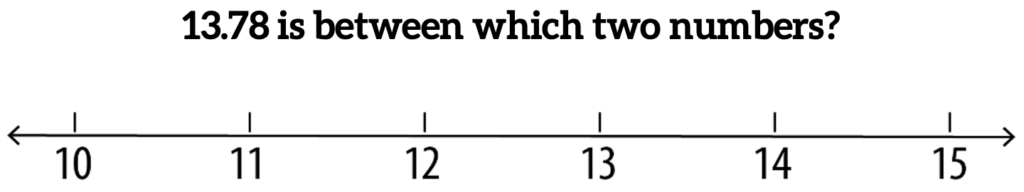
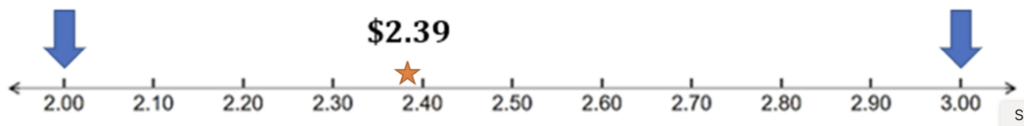
Proximity: Once students have located the number between two benchmarks, they need to understand which of the benchmarks it is closest to. For example, is $16.34 closer to $16 or $17? This is where the traditionally taught “5 and up” procedure might be applied by students decide if they should round up or down, since a 5 digit in the relevant place value is usually the halfway point between the two benchmarks. However, a number line can add a powerful visual to help students understand the concept of “closer to.”
Is $2.39 closer to $2 or $3?
The “5 and up” procedure is also not useful if we are rounding to benchmarks other than place values, for example, to the nearest $0.50. In that case, we want students to understand the idea of proximity and a half-way point. For example, $4.69 is closer to $4.50 than $5.00, because it has not yet reached the half-way point ($4.75).
Too high or too low: In real life applications of rounding, it is important to consider not only the closest benchmark, but also whether our rounded number will be higher or lower than the exact amount. In some situations, we may choose to round up no matter what; for example, if we are at the grocery store and want to avoid running out of money. Sometimes we prefer an over-or-under estimate to the closest possible one. As students are practicing rounding in different contexts, encourage them to both identify whether their estimate is above or below the exact amount, and if they prefer one or the other based on the situation.
Magnitude: Which benchmarks make sense? The magnitude of the number we are rounding, relative to the amount we are changing the number, makes a big difference. As we saw in the example with the age of the woman, rounding 48 years and 9 months to 49 years (nearest year) or 50 years (nearest ten) seemed reasonable and useful. Changing a number like 48 by a year or two is not very significant. However, rounding to the nearest 100 years (0!) was meaningless in that context. In the same way, it would be misleading to say that a 6.5-month-old baby is “almost one.” While it’s mathematically correct, it changes the age too much, relative to the age itself, to be useful. (This is also why saving $10 on lunch seems like a good deal, while saving $10 on a car is insignificant.)
Are you interested in teaching foundational concepts like rounding so your adult students can use them flexibly and authentically in their lives? Check out the Beginner Curriculum for Adults Learning Math (BeCALM) Curriculum units, available on the SABES Math Center resource page. BeCALM Part 1: Number Sense contains units and lessons on rounding and other estimation strategies. Also, check the SABES calendar for BeCALM workshops available to help you get started using these materials!

Melissa Braaten is an adult education instructor at Catholic Charities Haitian Multi-Services Center in Dorchester, MA. Melissa has taught ASE and pre-ASE math and reading, as well as ABE writing, computer skills, and health classes. Melissa also is a training and curriculum development specialist for the SABES Mathematics and Adult Numeracy Curriculum & Instruction PD Center at TERC. She is the author of many articles for Math Musings, our Adult Numeracy blog.
