In Defense of Guess-and-Check
by Sarah Lonberg-Lew
Problem solving is a lot more than reading a short story problem and performing one or two calculations. Real problem solving is what happens when you can’t see a clear path to a solution and have to be creative. There are many great problem-solving strategies: look for a pattern, examine a simpler case, draw a picture, model with an equation, etc. Also on this list is the humble guess-and-check, aka guess-check-and-revise. For some reason, when people learn fancier strategies like writing and solving equations, guess-and-check gets relegated to the status of beginner math and students are often in a hurry to leave it behind. It might be used as a last resort, but it isn’t real math – some students even see it as cheating. Guessing just isn’t as good as figuring something out. Even teachers sometimes see it as not real problem solving and pass on to students the unfortunate and discouraging message that they have just gotten lucky in solving the problem using guess-and-check but still need to learn a proper way of tackling the problem.
But guessing blindly and applying the strategy of guess-and-check are two completely different animals. When teachers and students dismiss guess-and-check as problem solving by luck, they are not seeing the sophisticated reasoning and understanding that must be brought to bear to use this approach which has as much right to be called a strategy as any other. Consider the following problem:
Tori has gotten the following scores on her last four math tests: 79, 86, 92, 88. What does she have to score on her fifth test to have an average score of 85?
Before you start writing an equation to find the answer, also consider why you might pose a problem like this to your students. What is it that you want to know about what they know? Is the important thing that they be able to abstract the definition of an average into symbols, or that they understand how averages behave and what they mean? Although it may be marginally faster to write and solve an equation (assuming one is already skilled at that), consider the reasoning and possibly even learning that can take place when you approach this with guess-and-check.
Let’s put ourselves in Tori’s shoes. She wants to get exactly an 85 average – no worse and no better. She decides to explore what will happen if she scores an 85 on the next test. This is reasonable because the scores are all fairly close to 85 already. Maybe she can hit the average by aiming right for it.
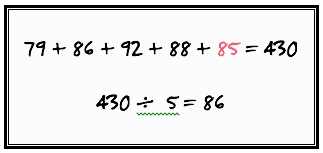
Testing out her guess, Tori adds up the five scores to get 430 and then divides by five to get an average of 86. That’s close! And Tori has just demonstrated that she knows how to find an average. Her guess got her close, but the answer was a little higher than what she was aiming for, so a second guess is needed.
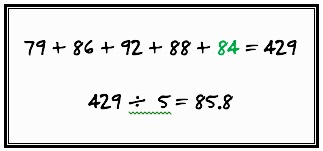
Because 85 was too high, Tori decides to try a lower number. She wants to lower the average by one, so she tries lowering the guess by one (that’s reasoning!). With a guess of 84, adding up the five scores gives a sum of 429 and dividing by five gets Tori to an average of 85.8.
Huh…. that didn’t result in the change she expected, but she also may have just discovered something about the structure of the situation and of averages in general – making a small change to one number makes an even smaller change to the average.
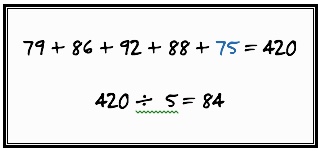
Next, Tori decides to try a much lower number. (Maybe if she can get by with a pretty low score, she can hang out with friends instead of studying the night before the test!) This time she tries 75. She arrives at a sum of 420 and an average of 84. Oops! That pushed the average too far in the other direction.
From here I’ll leave Tori to continue on her own. She knows now that 84 was too high and 75 was too low and I have confidence that she’ll hit the solution within a few more guesses. She’ll also have not only practiced with finding averages, but also seen how changing the numbers affects the average. She’ll have both made use of the structure of averages and deepened her understanding of it. She may have even noticed that the numbers she was trying contributed different amounts to the sum and if she wanted a sum that was going to give a result of 85 when divided by 5, there was a particular sum that she should be aiming for.
This is actually more thinking and learning than will be done by a student who knows how to model the situation by writing and solving an equation. There’s nothing wrong with that approach, either, but that student hasn’t really engaged in problem solving, only performed an exercise.
In order to use the strategy of guess-and-check, students must at least understand the structure of the problem. Without that understanding, they cannot check their guesses or make improved guesses. So, when they successfully navigate a problem with this strategy, neither they nor their teachers should chalk their success up to luck. Instead, students and teachers should appreciate the hard work and reasoning that goes into solving with guess-and-check as well as the learning that can result from it.
==================================================

Sarah Lonberg-Lew has been teaching and tutoring math in one form or another since college. She has worked with students ranging in age from 7 to 70, but currently focuses on adult basic education and high school equivalency. She teaches in an adult education program in Gloucester, MA. Sarah’s work with the SABES Mathematics and Adult Numeracy Curriculum & Instruction PD Center at TERC includes developing and facilitating trainings and assisting programs with curriculum development. She is the treasurer for the Adult Numeracy Network.
